The particular set of values. The definitions of sine cosine and tangent can be extended to the complex numbersby defining the functions by their Taylor seriesinstead of by the ratio of two lengths.

When To Use Restrictions Domain And Range On Trig Functions Mathematics Stack Exchange

Sin 1 x is an odd function and sin 1 x sin 1 x.

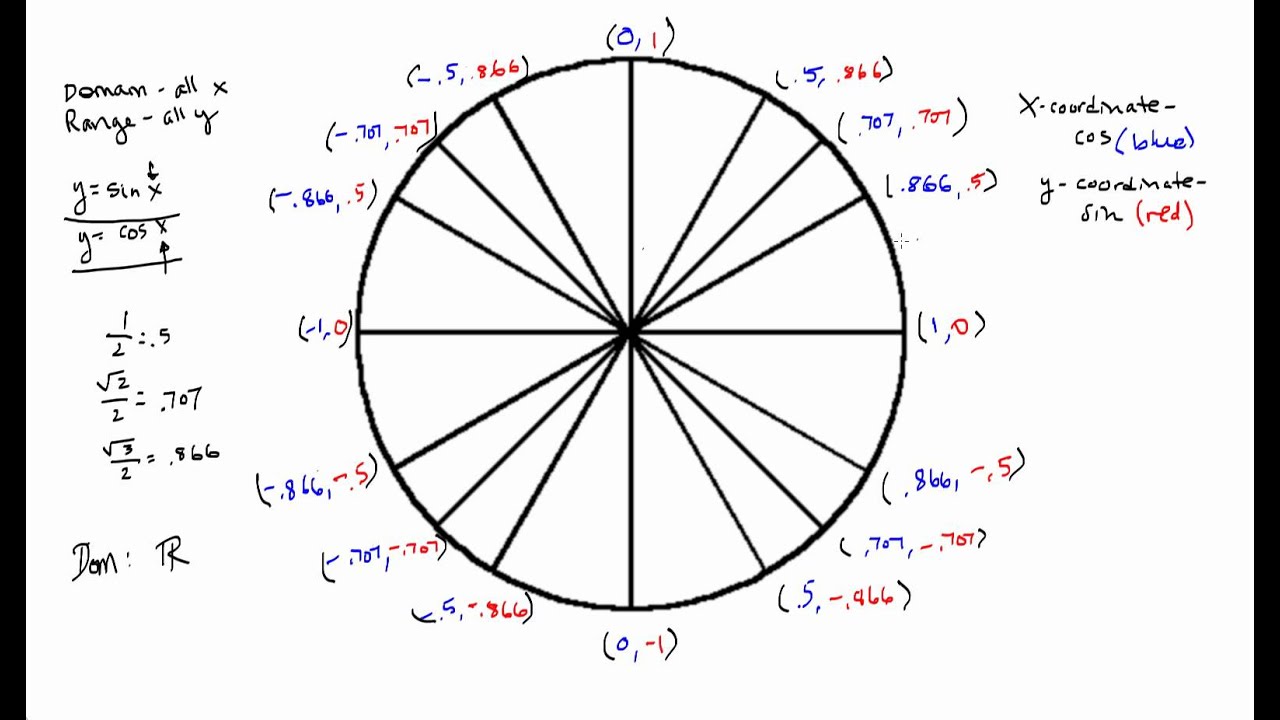
Domain of sine function. You can graphically represent all of the trigonometric functions. Range of sin x and cos x. The sine function takes the reals domain to the closed interval 11 1 1 range.

The domains of sine and cosine are infinite. R 1 1 Range. The sine of an acute angle is defined in the context of a right triangle.

Sinsin 1x x for x 2 11 sin 1sinx x for x 2 ˇ 2. For the function f x x the input value cannot be a negative number since the square root of a negative number is not a real number. Summary The sine is a function of trigonometry of an angle.

Since ff 1x x f 1fx x we have. Range of sin x. In the sine function the domain is all real numbers and the range is -1 to 1.

The set of values that can be used as inputs for the function is called the domain of the function. The sine functions have several distinct characteristics. 2ˇ 23ˇ 2g Range.

If you give each function an angle as input the domain is the possible range of values for the input you will get an output value the range. The shape of the sine curve is the same for each full rotation of the angle and so the function is called periodic. So the domain for sin x and cos x is all real numbers.

The period of the function is 360 or 2π radians. Plotting the points from the table and continuing along the x-axis gives the shape of the sine functionSee Figure PageIndex2. The sine of an acute angle can be defined with reference to a right.

F x sin x. More generally the definition of sine can be extended to any real value in terms of the length of a. Watch Domain and Range of Sine Cosine Functions in Hindi from Graphs of Trigonometric Functions and Domain and Range of Trigonometric Functions here.

These include the graph domain range asymptotes if any symmetry x and y intercepts and maximum and minimum points. The values of the sine function are different depending on whether the angle is in degrees or radians. The diagrams given below clearly explains the range of sin x and cos x.

The function fx sinx has all real numbers in its domain but its range is 1 sinx 1. The two trigonometric ratios sin x and cos x are defined for all real values of x. Here is the graph of the sine function.

Domain of inverse function Range of the function. Domainsin 1 ˇ11 and Rangesin ˇ 2. For the specified angle it is the ratio of the length of the side that is opposite that angle to the length of the longest side of the triangle.

The domain of each function is and the range is. Domain Range and Period of the three main trigonometric functions. Properties of sin 1 x.

Which means that theta can be any angle in degrees or radians any real number. If theta represents all the angles in the domain of the two functions. Sin 1 x y if and only if siny x and ˇ 2 y ˇ 2.

A mathematical function is like a black box that takes certain input values and generates corresponding output values. In that case the domain and range of the sine and cosine functions is all complex numbers and the domain of the tangent function is all complex numbers. Since f 1x y if and only if fy x.

Both of these functions can be extended so that their domains are the complex numbers and the ranges change as well. For an angle x displaystyle x the sine function is denoted simply as sin x displaystyle sin x. Watch all CBSE Class 5 to 12 Video Lectures here.

In trig speak you say something like this. ˇ Domain Range and De nition of the three main inverse trigonometric functions. Fxjx6 ˇ 2 kˇ k.

Not all functions work with all kinds of values. A range of a function is the set of output values for different input values. The function is periodic with periodicity 360 degrees or 2π radians.

So domain of sin-1x is -1 1 or -1 x 1 In the above table the range of all trigonometric functions are given. In mathematics the sine is a trigonometric function of an angle. The domain of the sine function As you drag the point A around notice that after a full rotation about B the graph shape repeats.

The sine function Notice how the sine values are positive between 0 and pi which correspond to the values of the sine function in quadrants I and II on the unit circle and the sine values are negative between pi and 2. They are periodic functions with a period of 2π.

This resource explains how to generate the graphs of sine cosine and tangent. They follow each other exactly π 2 radians 90 apart.

4 5a Graphs Of Sine And Cosine Functions Youtube

The graph has a period of 360.

How to graph sine and cosine. When we look at the graph that is generated by unwrapping our unit circle we will see that it generates a wave-like graph where the domain is all real numbers and the range is between -1 and 1. The sine and cosine graphs are very similar as they both. The three trigonometric ratios.

Thats the period of both functions 360 degrees. Being able to visualize the trigonometric functions is important. The graph of y sintheta has a maximum value of 1 and a minimum value of -1.

For a sine or cosine graph simply go from 0 to 2π on the x-axis and -1 to 1 on the y-axis intersecting at the origin 0 0. In the general formula for a sinusoidal function the period is latextextPfrac2piBlatex. Y cos x displaystyle ycos x.

Y sin x displaystyle ysin x and. This is due to the fact that as we move around the unit circle in a counterclockwise fashion we notice that the largest value for sine or cosine is 1 therefore its amplitude height is also 1 as nicely shown by Khan Academy. Quarter 2 - Module 3.

Draw a coordinate plane. Have the same curve only shifted along the x-axis have an amplitude half the distance between the maximum and minimum values of 1 have a. Top tip for the exam.

This video will get you up to speed by showing you the basic graph for sine and cosine. Sine cosine and tangent are used to calculate angles and lengths in right-angled triangles. This trigonometry and precalculus video tutorial shows you how to graph trigonometric functions such as sine and cosine functions using transformations phas.

First note that the sine and cosine graphs are the same shape cosine is the same as sine just slid 90 degrees to the left. This means that it repeats itself every 360. Cosine is just like Sine but it starts at 1 and heads down until π radians 180 and then heads up again.

Graphs of Circular FunctionsPart 1 - Sine and Cosine FUnctionsBackgro. Plot of Sine and Cosine In fact Sine and Cosine are like good friends. It includes pupil worksheets used in the powerpoint in word and PDF form.

How to graph them when the amplitude and period change. Plot of the Tangent Function. If C 0 the graph shifts to the left.

If C 0 the graph shifts to the right. Y AsinBx C B D and y AcosBx C B D. It also goes on to look at translations and reflections of the trig functions.

It used the unit circle to help explain this. The graph of y cos θ. The value C B for a sinusoidal function is called the phase shift or the horizontal displacement of the basic sine or cosine function.

Sine and cosine graphs y sin x and y cos x look pretty similar. Visualizing the Amplitude of the Sine Function. The graph of a sinusoidal function has the same general shape as a sine or cosine function.

In fact the main difference is that the sine graph starts at 00 and the cosine at 01. We discuss how to find the amplitude period phase shift and vertical shift and look at tips for making gr. We discuss how to graph Sine and Cosine graphs.

The sine and cosine rules calculate lengths and angles in any triangle. In the general formula for a sinusoidal function A represents amplitude. To check youve drawn the right one simply use your calculator to find sin 0 which is 0 or cos 0 which is 1 to make sure youre starting in the right place.

Also notice that their simple wave shape goes as high as 1 and as low as 1 and goes on forever to the left and right repeating every 360 degrees.

Notice that the output of each of these inverse functions is a number an angle in radian measure. The graphs of the inverse functions are shown in Figure 4 Figure 5 and Figure 6.

Inverse Trigonometric Functions

Therefore the inverse of secant function can be expressed as.

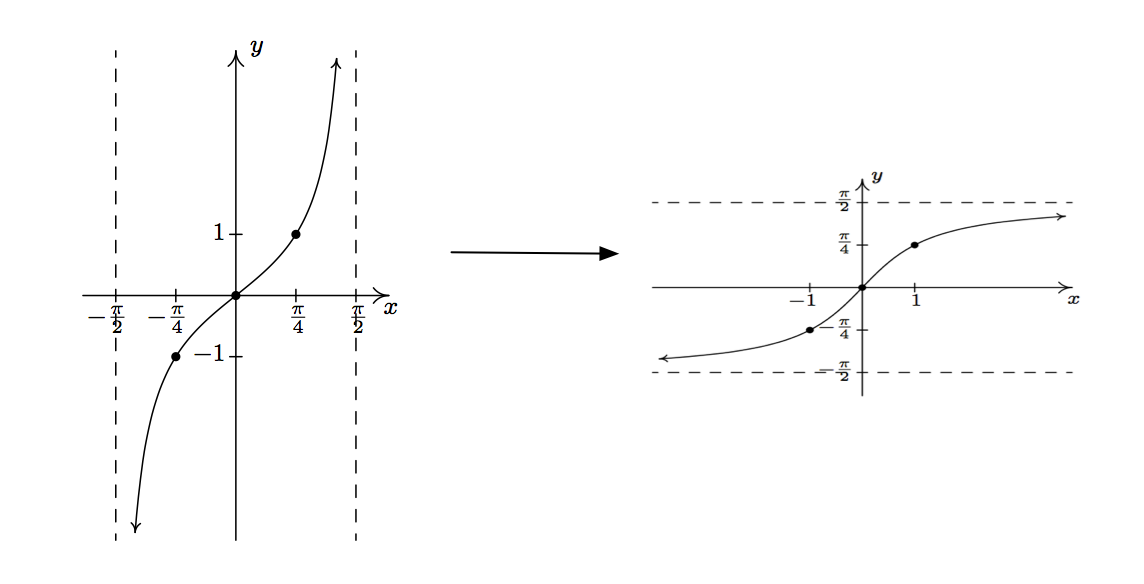
Graph of inverse sine function. Now for its inverse to also be a function it must pass the horizontal line test. Consider the graph of the function. The vertical lines represent the restricted domain of the sin function.

It is represented in the graph as shown below. Inverse of Sine Function y sin-1 x sin-1 x is the inverse function of sinx. It starts at 0 heads up to 1 by π 2 radians 90 and then heads down to 1.

Explain whether or not the complete inverse of fx sinx is a function. Consider the graph of the function fx sinx shown below. Let us recall that sine function is a function with R as its domain and 1 1 as its range.

The figure shows what the graphs of inverse sine and cosine look like. In this article we will learn about graphs and nature of various inverse functions. This means that is a function.

In mathematics the inverse trigonometric functions occasionally also called arcus functions antitrigonometric functions or cyclometric functions are the inverse functions of the trigonometric functions with suitably restricted domainsSpecifically they are the inverses of the sine cosine tangent cotangent secant and cosecant functions and are used to obtain an angle from any of. In radians thats - π 2 π 2. Its domain is 1 1 and its range is - π2 π2.

We know that trig functions are especially applicable to the right angle triangle. Sin-1 Opposite side hypotenuse θ. Here the symbol 1 is not an exponent.

The Inverse Sine Function arcsin We define the inverse sine function as yarcsin x for -pi2. To graph the inverse sine function we first need to limit or more simply pick a portion of our sine graph to work with. Using coordinate points of the graph to assist you and create a sketch of the inverse of fx sinx.

- x -1 or 1 x. The Sine Function has this beautiful up-down curve which repeats every 2 π radians or 360. This means that if a horizontal line is drawn anywhere on the graph it will only pass through one point.

The inverse sine function formula or the arcsin formula is given as. In approximate decimal values that range is 0 to 3142. Use online calculator for trigonometry.

Inverse sine has a domain of -1 1 and a range of -π 2 π 2. Use the sliders to adjust the parameters of the sin function. To find the inverse sine graph we need to swap the variables.

The inverse trigonometric functions actually perform the opposite operation of the trigonometric functions such as sine cosine tangent cosecant secant and cotangent. The graphs of the inverse functions are the original function in the domain specified above which has been flipped about the line y x yx y x. Sec 33 Basic Trigonometric Equations Inverses of Trigonometric Functions Name.

X becomes y and y becomes x. Plot of Sine. This means that x sin y The graph of y arcsin x.

-π2 y π2. Graph of Inverse Sine Function. As you do this note how the graph of the inverse sin function changes.

These points are the extreme values of the inputs. We see that sin1x sin 1 x has domain 1 1 and range π 2 π 2 π 2 π 2 cos1x cos 1. Next we limit the domain to -90 90.

Heres the graph of the inverse sine function y sin-1 x or y arcsin x. Ddx Sin-1 x 1 1-x 2. It intersects the coordinate axis at 00.

It passes the vertical line test that is if a vertical line is drawn anywhere on the graph it only passes through a single point of the function. The effect of flipping the graph about the line y x yx y x is to swap the roles of x x x and y y y so this observation is true for the graph of any inverse function. Lets see the graph of y sin x first and then derive the curve of y arcsin x.

Sine Function and Inverse Sine Function. Arcsecant function is the inverse of the secant function denoted by sec -1x. It denotes the inverse and does not mean the reciprocal.

We write y sin x and y sin 1 x or y arcsinx to represent the sine function and the inverse sine function respectively. The red dashed line represents the line of reflection for the sin graph and its inverse. Introduction to Inverse Trig Function.

Arcsine trigonometric function is the sine function is shown as sin-1 a and is shown by the below graph. Heres the graph of y sin x. The points indicated on the graphs are at x 1 and x 1.

The derivative of inverse sine function is given by. Y sec-1x arcsecant x Domain Range of Arcsecant. See how the angles are on the y-axis this time instead of on the x-axis like they were for the sine function.

The graphs of y sin 1 x and y cos 1 x. Inverse trigonometric function graphs for sine cosine tangent cotangent secant and cosecant as a function of values.