In this article we will learn what a domain and range of a function mean and how to calculate the two quantities. The domain of f above is the set of all values of x in the interval - As x takes values from - to sin x takes all values between -1 and 1 as shown in the unit circle below.
The sine functions have several distinct characteristics.
Domain and range of a sine function. To find the range of a function first find the x value and y value of the vertex using the formula x b 2a. Introduction to Trigonometric Functions. The sine function takes the reals domain to the closed interval range.
Browse more Topics Under Trigonometric Functions. The values taken by the function are collectively referred to as the range. R 1 1 Range.
Watch all CBSE Class 5 to 12 Video Lectures here. We also know that for each real number x-1 sinx 1 and -1 cosx 1. Informally if a function is defined on some set then we call that set the domain.
The sine and cosine functions are unique in the world of trig functions because their ratios always have a value. The range is the resulting values that the dependant variable can have as x varies throughout the domain. That is range of sinx is -1 1 And also we know the fact Domain of inverse function Range of the function.
Domain and range for sine and cosine functions There are no restrictions on the domain of sine and cosine functions. For example the function takes the reals domain to the non-negative reals range. These include the graph domain range asymptotes if any symmetry x and y intercepts and maximum and minimum points.
Now let us discuss the function fx tan x. Domain and Range of Sine and Cosine Functions. Before getting into the topic of domain and range lets briefly describe what a function is.
Domain and Range of a Function Explanation Examples. The domain of each function is and the range is 11. By Mary Jane Sterling.
Key Point The function fx sinx has all real numbers in its domain but its range is 1 sinx 1. Domain of a function. So the domain for sin x and cos x is all real numbers.
Notice however that the range for both y sinx and y cosx is between -1 and 1. Hence for the trigonometric functions fx sin x and fx cos x the domain will consist of the entire set of real numbers as they are defined for all the real numbers. Therefore their domain is such that x R.
Domain and Range of Trigonometric Functions. So domain of sin-1 x is -1 1 or -1 x 1. Project supervised by Dr.
Let the constant function be f x k. No matter what angle you input you get a resulting output. The hyperbolic functions take a real argument called a hyperbolic angleThe size of a hyperbolic angle is twice the area of its hyperbolic sectorThe hyperbolic functions may be defined in terms of the legs of a right triangle covering this sector.
The range of fx sin x and fx cos x will lie from -1 to 1 including both -1 and 1 ie-1 sin x 1-1 cos x 1. Kˇ k. It is the set x in the notation f.
The value you get may be 0 but thats a number too. The function is periodic with periodicity 360 degrees or 2π radians. In the above six trigonometric ratios the first two trigonometric ratios sin x and cos x are defined for all real values of x.
This video will show you how to find domain and range of trigonometric functions. Domain of Inverse Trigonometric Functions. The two trigonometric ratios sin x and cos x are defined for all real values of x.
Range of sin x and cos x. Already we know the range of sinx. Both of these functions.
Watch Domain and Range of Sine Cosine Functions in Hindi from Graphs of Trigonometric Functions and Domain and Range of Trigonometric Functions here. They are periodic functions with a period of 2π. -1 sin x 1 or -1 y 1.
The graph of y sin x is symmetric about the origin because its an odd function. Sin x cos x csc x sec x tan x cot x. Domain Range and Period of the three main trigonometric functions.
F x sin x. In general the range of any sine function of the form y sin b x c is given by. In complex analysis the hyperbolic functions arise as the imaginary parts of sine and cosineThe hyperbolic sine and the hyperbolic cosine are.
The range of a function is the set of all the outputs a function can give. We know that the sine and cosine functions are defined for all real numbers. The values of the sine function are different depending on whether the angle is in degrees or radians.
Click the File tab and select Open or press Ctrl O. Your Excel formulas cheat sheet.
These formulas we can use in Excel 2013.
Excel function cheat sheet. Use this handy Cheat Sheet to discover great functions and tips to help you get the most out of Excel. The LEFT function can return text from the beginning of a cell left to right MID returns text from any start point of the cell left to right and RIGHT returns text from the end of the cell right to left. STATISTICAL Functions in Excel.
Some Excel Formulas Cheat Sheet is given below. Excel Cheat Sheet Basic Skills The Excel Program Screen Keyboard Shortcuts Getting Started Create a Workbook. 15 tips for calculations and common tasks Many of us fell in love with Excel as we delved into its deep and sophisticated formula features.
If you have trouble viewing these PDFs install the free Adobe Acrobat Reader DC. Enter cell references with pointing. Visual Basic for Applications makes automation possible in Excel and other Office applications.
Select the cell where you want to insert the formula. The above-explained cheat sheet of excel formulas examples is implemented in the excel worksheet shown in the following screenshot. The following formulas apply to Excel 2017.
Excel Formulas PDF is a list of most useful or extensively used excel formulas in day to day working life with Excel. MS Excel offers a variety of date and time functions. The table contains the name of the function the meaning of the function the syntax and the example.
Go to FORMULA tab. Nov 12 2018 - Without formulas Excel is just a word processor. The following list shows an array of Excel functions used by one and all.
Click on TEXT functions. SUM All Excel formula. Calculations used in Cheat Sheet of Excel Formulas TEXT Functions in Excel.
To create a formula with references highlight the cells you want to use then select an empty cell and enter the formula. Excel Function Cheatsheets The key to being efficient with MS Excel is knowing which functions to use. Instead of writing the whole formula click on to the cell in which you want your formula to be placed and then click on to Insert Function option under the Formulas tab.
Go to FORMULAS tab. The below Excel VBA Cheatsheet is your one stop shop for a variety of useful VBA automations. The CELL function can return a variety of information about the contents of a cell such as its name location row column and more.
In the Insert Function dialogue box select Math Trig and under the Select a function drop-down menu select SUM and press OK. People say if you learn 20-25 formulas you can master Microsoft Excel. This formula is useful when working with Excel functions that have a date as.
Excel formulas cheat sheet Shortcuts. Download my Excel workbook where I have listed 100 common Microsoft Excel Formulas and Functions Cheat Sheet for your daily use. Select a recent file or navigate to the location where the file is saved.
Choose Statistical Functions category. VLOOKUP INDEX MATCH RANK AVERAGE SMALL LARGE LOOKUP ROUND COUNTIFS SUMIFS FIND DATE and many more. Get up to speed in minutes quickly refer to things youve learned and master keyboard shortcuts.
If you are new to VBA start with my Excel VBA Tutorial. Click on More Functions option. The Excel Functions covered here are.
Start the formula with an sign select a cell enter an operator like or then select another cell. With the cheatsheets below you can find functions for working with finance statistics and database content among others. Click the Insert Function button Use the Insert Function button under the Formulas tab to select a function from Excels menu list.
If youre using a slightly older version of Excel the location of each feature mentioned below might be slightly different. Dates and time Excel formulas cheat sheet. Without Formulas Microsoft Excel is just like a word processor.
Because there are multiple ways to get results you can decide which method works best for you. Type and enter the formula using values cell references operators and functions. Excel adds up this list you just pointed to as B4B5B6B7.
For example there are several ways to enter formulas and calculate numbers. Excel Functions Youre Likely to Use Some Excel functions apply to specific subject areas but others are general and apply to all needs. Navigation Excel Formulas.
2016 as well as 2019. Excel Cheat Sheet Keyboard shortcuts in Excel NOTE. A drop-down list will open.
Click the File tab and select New or press Ctrl N. Free download a cheat sheet with 100 common Microsoft Excel Formulas and Functions for your daily use. Be sure to bookmark this page as your Excel VBA cheat sheet.
These are Excel formulas and functions that give life to the worlds most popular spreadsheet program. Excel Shortcuts PC Mac Excel Shortcuts - List of the most important common MS Excel shortcuts for PC Mac.
The particular set of values. The definitions of sine cosine and tangent can be extended to the complex numbersby defining the functions by their Taylor seriesinstead of by the ratio of two lengths.
Sin 1 x is an odd function and sin 1 x sin 1 x.
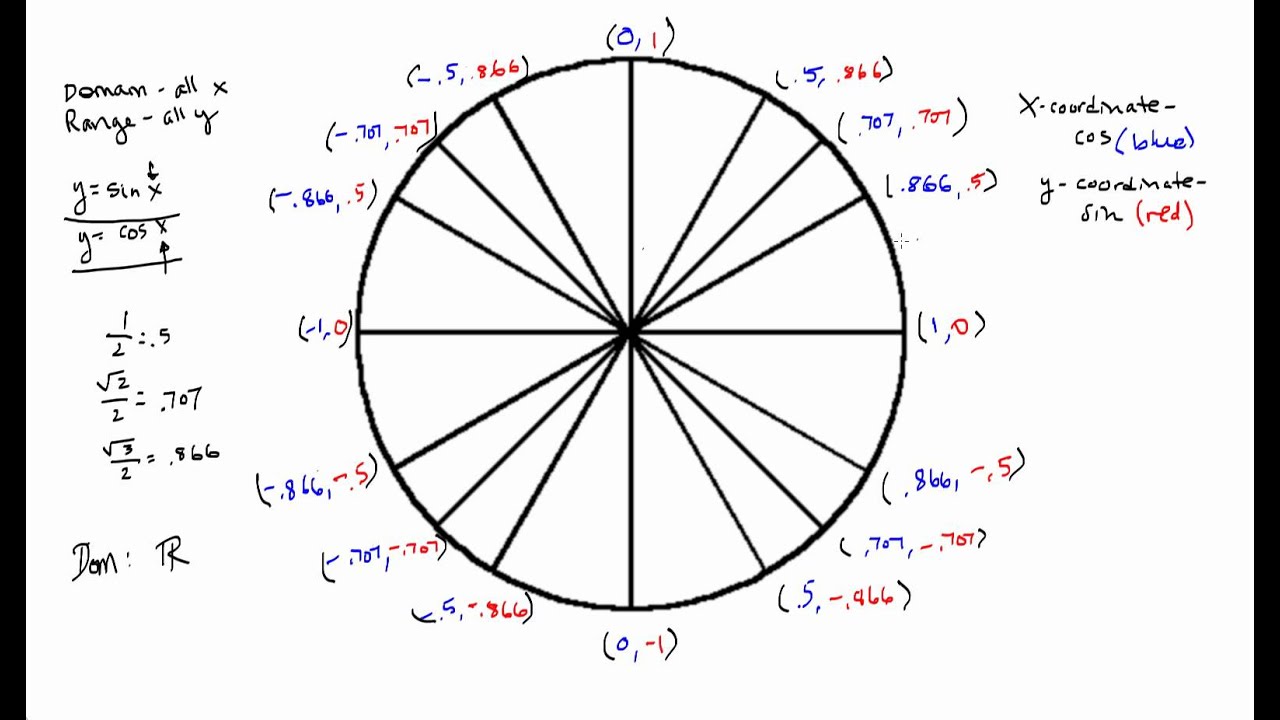
Domain of sine function. You can graphically represent all of the trigonometric functions. Range of sin x and cos x. The sine function takes the reals domain to the closed interval 11 1 1 range.
The domains of sine and cosine are infinite. R 1 1 Range. The sine of an acute angle is defined in the context of a right triangle.
Sinsin 1x x for x 2 11 sin 1sinx x for x 2 ˇ 2. For the function f x x the input value cannot be a negative number since the square root of a negative number is not a real number. Summary The sine is a function of trigonometry of an angle.
Since ff 1x x f 1fx x we have. Range of sin x. In the sine function the domain is all real numbers and the range is -1 to 1.
The set of values that can be used as inputs for the function is called the domain of the function. The sine functions have several distinct characteristics. 2ˇ 23ˇ 2g Range.
If you give each function an angle as input the domain is the possible range of values for the input you will get an output value the range. The shape of the sine curve is the same for each full rotation of the angle and so the function is called periodic. So the domain for sin x and cos x is all real numbers.
The period of the function is 360 or 2π radians. Plotting the points from the table and continuing along the x-axis gives the shape of the sine functionSee Figure PageIndex2. The sine of an acute angle can be defined with reference to a right.
F x sin x. More generally the definition of sine can be extended to any real value in terms of the length of a. Watch Domain and Range of Sine Cosine Functions in Hindi from Graphs of Trigonometric Functions and Domain and Range of Trigonometric Functions here.
These include the graph domain range asymptotes if any symmetry x and y intercepts and maximum and minimum points. The values of the sine function are different depending on whether the angle is in degrees or radians. The diagrams given below clearly explains the range of sin x and cos x.
The function fx sinx has all real numbers in its domain but its range is 1 sinx 1. The two trigonometric ratios sin x and cos x are defined for all real values of x. Here is the graph of the sine function.
Domain of inverse function Range of the function. Domainsin 1 ˇ11 and Rangesin ˇ 2. For the specified angle it is the ratio of the length of the side that is opposite that angle to the length of the longest side of the triangle.
The domain of each function is and the range is. Domain Range and Period of the three main trigonometric functions. Properties of sin 1 x.
Which means that theta can be any angle in degrees or radians any real number. If theta represents all the angles in the domain of the two functions. Sin 1 x y if and only if siny x and ˇ 2 y ˇ 2.
A mathematical function is like a black box that takes certain input values and generates corresponding output values. In that case the domain and range of the sine and cosine functions is all complex numbers and the domain of the tangent function is all complex numbers. Since f 1x y if and only if fy x.
Both of these functions can be extended so that their domains are the complex numbers and the ranges change as well. For an angle x displaystyle x the sine function is denoted simply as sin x displaystyle sin x. Watch all CBSE Class 5 to 12 Video Lectures here.
In trig speak you say something like this. ˇ Domain Range and De nition of the three main inverse trigonometric functions. Fxjx6 ˇ 2 kˇ k.
Not all functions work with all kinds of values. A range of a function is the set of output values for different input values. The function is periodic with periodicity 360 degrees or 2π radians.
So domain of sin-1x is -1 1 or -1 x 1 In the above table the range of all trigonometric functions are given. In mathematics the sine is a trigonometric function of an angle. The domain of the sine function As you drag the point A around notice that after a full rotation about B the graph shape repeats.
The sine function Notice how the sine values are positive between 0 and pi which correspond to the values of the sine function in quadrants I and II on the unit circle and the sine values are negative between pi and 2. They are periodic functions with a period of 2π.
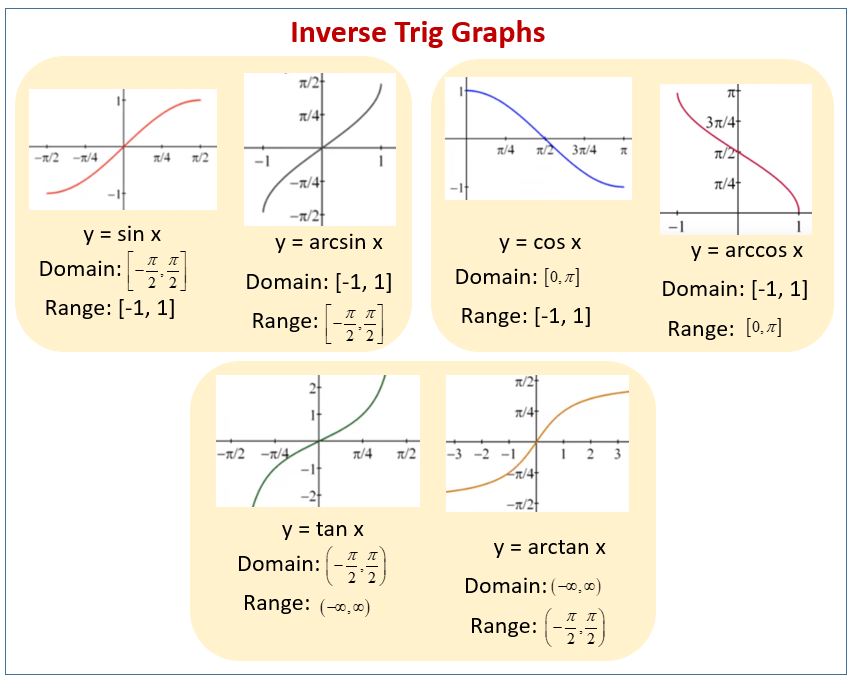
Notice that the output of each of these inverse functions is a number an angle in radian measure. The graphs of the inverse functions are shown in Figure 4 Figure 5 and Figure 6.
Therefore the inverse of secant function can be expressed as.
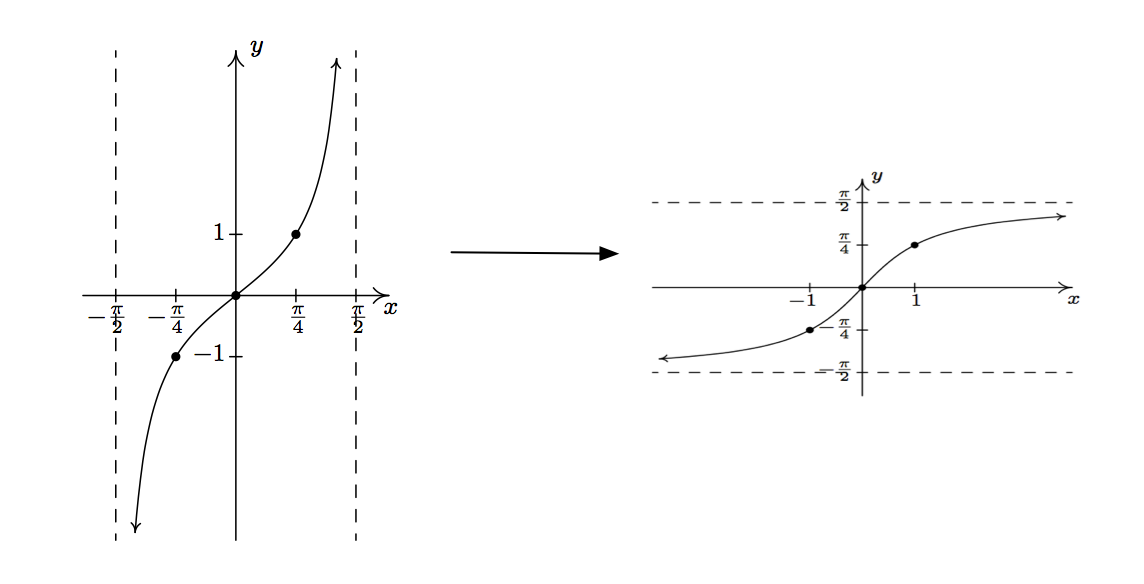
Graph of inverse sine function. Now for its inverse to also be a function it must pass the horizontal line test. Consider the graph of the function. The vertical lines represent the restricted domain of the sin function.
It is represented in the graph as shown below. Inverse of Sine Function y sin-1 x sin-1 x is the inverse function of sinx. It starts at 0 heads up to 1 by π 2 radians 90 and then heads down to 1.
Explain whether or not the complete inverse of fx sinx is a function. Consider the graph of the function fx sinx shown below. Let us recall that sine function is a function with R as its domain and 1 1 as its range.
The figure shows what the graphs of inverse sine and cosine look like. In this article we will learn about graphs and nature of various inverse functions. This means that is a function.
In mathematics the inverse trigonometric functions occasionally also called arcus functions antitrigonometric functions or cyclometric functions are the inverse functions of the trigonometric functions with suitably restricted domainsSpecifically they are the inverses of the sine cosine tangent cotangent secant and cosecant functions and are used to obtain an angle from any of. In radians thats - π 2 π 2. Its domain is 1 1 and its range is - π2 π2.
We know that trig functions are especially applicable to the right angle triangle. Sin-1 Opposite side hypotenuse θ. Here the symbol 1 is not an exponent.
The Inverse Sine Function arcsin We define the inverse sine function as yarcsin x for -pi2. To graph the inverse sine function we first need to limit or more simply pick a portion of our sine graph to work with. Using coordinate points of the graph to assist you and create a sketch of the inverse of fx sinx.
- x -1 or 1 x. The Sine Function has this beautiful up-down curve which repeats every 2 π radians or 360. This means that if a horizontal line is drawn anywhere on the graph it will only pass through one point.
The inverse sine function formula or the arcsin formula is given as. In approximate decimal values that range is 0 to 3142. Use online calculator for trigonometry.
Inverse sine has a domain of -1 1 and a range of -π 2 π 2. Use the sliders to adjust the parameters of the sin function. To find the inverse sine graph we need to swap the variables.
The inverse trigonometric functions actually perform the opposite operation of the trigonometric functions such as sine cosine tangent cosecant secant and cotangent. The graphs of the inverse functions are the original function in the domain specified above which has been flipped about the line y x yx y x. Sec 33 Basic Trigonometric Equations Inverses of Trigonometric Functions Name.
X becomes y and y becomes x. Plot of Sine. This means that x sin y The graph of y arcsin x.
-π2 y π2. Graph of Inverse Sine Function. As you do this note how the graph of the inverse sin function changes.
These points are the extreme values of the inputs. We see that sin1x sin 1 x has domain 1 1 and range π 2 π 2 π 2 π 2 cos1x cos 1. Next we limit the domain to -90 90.
Heres the graph of the inverse sine function y sin-1 x or y arcsin x. Ddx Sin-1 x 1 1-x 2. It intersects the coordinate axis at 00.
It passes the vertical line test that is if a vertical line is drawn anywhere on the graph it only passes through a single point of the function. The effect of flipping the graph about the line y x yx y x is to swap the roles of x x x and y y y so this observation is true for the graph of any inverse function. Lets see the graph of y sin x first and then derive the curve of y arcsin x.
Sine Function and Inverse Sine Function. Arcsecant function is the inverse of the secant function denoted by sec -1x. It denotes the inverse and does not mean the reciprocal.
We write y sin x and y sin 1 x or y arcsinx to represent the sine function and the inverse sine function respectively. The red dashed line represents the line of reflection for the sin graph and its inverse. Introduction to Inverse Trig Function.
Arcsine trigonometric function is the sine function is shown as sin-1 a and is shown by the below graph. Heres the graph of y sin x. The points indicated on the graphs are at x 1 and x 1.
The derivative of inverse sine function is given by. Y sec-1x arcsecant x Domain Range of Arcsecant. See how the angles are on the y-axis this time instead of on the x-axis like they were for the sine function.
The graphs of y sin 1 x and y cos 1 x. Inverse trigonometric function graphs for sine cosine tangent cotangent secant and cosecant as a function of values.
ads
quizzes for kids age 5
Quiz for 5 year olds Quizzy Kid . Quizzes by age. Quiz for 5 year olds. Quiz for 5 year olds Quiz for 5...
